Trigonometric inequalities. Trigonometric inequalities and their solutions How to solve double trigonometric inequalities
Ministry of Education of the Republic of Belarus
educational institution
"Gomel State University
named after Francysk Skaryna"
Faculty of Mathematics
Department of Algebra and Geometry
Eligible for defense
Head Department Shemetkov L.A.
Trigonometric equations and inequalities
Course work
Executor:
student group M-51
CM. Gorsky
Scientific adviser
Senior Lecturer
V.G. Safonov
Gomel 2008
INTRODUCTION
BASIC METHODS FOR SOLVING TRIGONOMETRIC EQUATIONS
Factorization
Solving equations by converting the product of trigonometric functions into a sum
Solving Equations Using Triple Argument Formulas
Multiplication by some trigonometric function
NON-STANDARD TRIGONOMETRIC EQUATIONS
TRIGONOMETRIC INEQUALITIES
SELECTION OF ROOTS
TASKS FOR INDEPENDENT SOLUTION
CONCLUSION
LIST OF USED SOURCES
In ancient times, trigonometry arose in connection with the needs of astronomy, surveying and construction, that is, it was purely geometric in nature and represented mainly<<исчисление хорд>>. Over time, some analytical points began to intersperse into it. In the first half of the 18th century there was a sharp turn, after which trigonometry took a new direction and shifted towards mathematical analysis. It was at this time that trigonometric dependencies began to be considered as functions.
Trigonometric equations are one of the most difficult topics in the school mathematics course. Trigonometric equations arise when solving problems in planimetry, solid geometry, astronomy, physics and other areas. Trigonometric equations and inequalities from year to year are found among the tasks of centralized testing.
The most important difference between trigonometric equations and algebraic ones is that algebraic equations have a finite number of roots, while trigonometric equations have an infinite number, which greatly complicates the selection of roots. Another specificity of trigonometric equations is the non-unique form of writing the answer.
This thesis is devoted to methods for solving trigonometric equations and inequalities.
The diploma work consists of 6 sections.
The first section contains the basic theoretical information: the definition and properties of trigonometric and inverse trigonometric functions; table of values of trigonometric functions for some arguments; expression of trigonometric functions in terms of other trigonometric functions, which is very important for converting trigonometric expressions, especially those containing inverse trigonometric functions; in addition to the basic trigonometric formulas, well known from the school course, formulas are given that simplify expressions containing inverse trigonometric functions.
The second section outlines the main methods for solving trigonometric equations. The solution of elementary trigonometric equations, the method of factoring, methods of reducing trigonometric equations to algebraic ones are considered. In view of the fact that the solutions of trigonometric equations can be written in several ways, and the form of these solutions does not allow one to immediately establish whether these solutions are the same or different, which can<<сбить с толку>> when solving tests, a general scheme for solving trigonometric equations is considered and the transformation of groups of general solutions of trigonometric equations is considered in detail.
The third section deals with non-standard trigonometric equations, the solutions of which are based on the functional approach.
The fourth section deals with trigonometric inequalities. Methods for solving elementary trigonometric inequalities, both on a unit circle and by a graphical method, are considered in detail. The process of solving non-elementary trigonometric inequalities through elementary inequalities and the method of intervals already well known to schoolchildren are described.
The fifth section presents the most difficult tasks: when it is necessary not only to solve a trigonometric equation, but also to select roots from the found roots that satisfy some condition. This section provides solutions to typical tasks for the selection of roots. The necessary theoretical information for the selection of roots is given: the partition of the set of integers into non-intersecting subsets, the solution of equations in integers (diophantine).
The sixth section presents tasks for independent solution, designed in the form of a test. The 20 test tasks list the most difficult tasks that can be encountered in centralized testing.
Elementary trigonometric equations
Elementary trigonometric equations are equations of the form , where is one of the trigonometric functions: , , , .
Elementary trigonometric equations have infinitely many roots. For example, the following values satisfy the equation: , , , etc. The general formula by which all the roots of the equation are found, where , is:
Here it can take any integer values, each of them corresponds to a certain root of the equation; in this formula (as well as in other formulas by which elementary trigonometric equations are solved) is called parameter. They usually write down, thereby emphasizing that the parameter can take any integer values.
Solutions of the equation , where , are found by the formula
The equation is solved by applying the formula
and the equation --- according to the formula
Let us especially note some special cases of elementary trigonometric equations, when the solution can be written without using general formulas:
When solving trigonometric equations, the period of trigonometric functions plays an important role. Therefore, we present two useful theorems:
Theorem If --- the main period of the function, then the number is the main period of the function.
The periods of the functions and are called commensurable if there are natural numbers and , that .
Theorem If periodic functions and , have commensurate and , then they have a common period , which is the period of the functions , , .
The theorem says what is the period of the function , , , and is not necessarily the main period. For example, the main period of the functions and is --- , and the main period of their product is --- .
Introducing an Auxiliary Argument
The standard way of converting expressions of the form 
is the following trick: let --- the angle given by the equalities 
, 
. For any and such an angle exists. In this way . If , or , , , otherwise .
Scheme for solving trigonometric equations
The main scheme that we will be guided by when solving trigonometric equations is as follows:
the solution of the given equation is reduced to the solution of elementary equations. Solutions --- transformations, factorizations, replacement of unknowns. The guiding principle is not to lose roots. This means that when moving to the next equation (equations), we are not afraid of the appearance of extra (extraneous) roots, but we only care that each subsequent equation of our "chain" (or a set of equations in the case of branching) is a consequence of the previous one. One possible method for selecting roots is to check. We note right away that in the case of trigonometric equations, the difficulties associated with the selection of roots, with verification, as a rule, increase sharply in comparison with algebraic equations. After all, you have to check the series, consisting of an infinite number of members.
Special mention should be made of the change of unknowns in solving trigonometric equations. In most cases, after the necessary replacement, an algebraic equation is obtained. Moreover, equations are not so rare, which, although they are trigonometric in appearance, are essentially not so, since already after the first step --- changes of variables --- turn into algebraic ones, and the return to trigonometry occurs only on stage of solving elementary trigonometric equations.
Recall once again: the replacement of the unknown should be done as soon as possible, the resulting equation after the replacement must be solved to the end, including the stage of selecting roots, and only then will it return to the original unknown.
One of the features of trigonometric equations is that the answer in many cases can be written in various ways. Even to solve the equation 
the response can be written like this:
1) in the form of two series: 
, , ;
2) in standard form, which is a union of the above series: , ;
3) because 
, then the answer can be written as 
, . (Further on, the presence of the parameter , , or in the response record automatically means that this parameter takes all possible integer values. Exceptions will be stipulated.)
Obviously, the three listed cases do not exhaust all the possibilities for writing the answer to the equation under consideration (there are infinitely many of them).
For example, for 
. Therefore, in the first two cases, if , we can replace by .
Usually, the answer is written on the basis of paragraph 2. It is useful to remember the following recommendation: if the work does not end with the solution of the equation, it is still necessary to conduct a study, the selection of roots, then the most convenient form of recording is indicated in paragraph 1. (A similar recommendation should be given for the equation.)
Let's consider an example illustrating what has been said.
Example Solve the equation.
Solution. The most obvious is the following way. This equation splits into two: and . Solving each of them and combining the answers obtained, we find .
Another way. Since , then, replacing and by the reduction formulas. After minor transformations, we get , whence 
.
At first glance, the second formula has no particular advantages over the first. However, if we take, for example, , then it turns out that , i.e. the equation has a solution, while the first way leads us to the answer 
. "See" and prove equality 
not so easy.
Answer. .
Transformation and union of groups of general solutions of trigonometric equations
We will consider an arithmetic progression that extends indefinitely in both directions. The terms of this progression can be divided into two groups of terms, located to the right and to the left of a certain term, called the central or zero term of the progression.
Fixing one of the terms of the infinite progression with a zero number, we will have to carry out a double numbering for all the remaining terms: positive for the terms located to the right, and negative for the terms located to the left of zero.
In the general case, if the difference of the progression is the zero term, the formula for any (th) term of the infinite arithmetic progression is:
Formula transformations for any member of an infinite arithmetic progression
1. If we add or subtract the difference of the progression to the zero term, then the progression will not change from this, but only the zero term will move, i.e. the numbering of the members will change.
2. If the coefficient of a variable is multiplied by , then this will only result in a permutation of the right and left groups of terms.
3. If successive members of an infinite progression
for example , , , ..., , to make the central terms of progressions with the same difference equal to :
then the progression and the series of progressions express the same numbers.
Example The row can be replaced by the following three rows: , , .
4. If infinite progressions with the same difference have numbers as central members that form an arithmetic progression with a difference , then these series can be replaced by one progression with a difference , and with a central member equal to any of the central members of these progressions, i.e. if
then these progressions are combined into one:
Example
, , , both are combined into one group, since 
.
To transform groups that have common solutions into groups that do not have common solutions, these groups are decomposed into groups with a common period, and then we try to combine the resulting groups, excluding repeating ones.
Factorization
The factorization method is as follows: if
then any solution of the equation
is the solution of the set of equations
The converse statement is, generally speaking, false: not every solution of the set is a solution to the equation. This is due to the fact that the solutions of individual equations may not be included in the domain of definition of the function .
Example Solve the equation.
Solution. Using the basic trigonometric identity, we represent the equation in the form
Answer.
; 
.
Converting the sum of trigonometric functions to a product
Example
solve the equation 
.
Solution. We apply the formula, we obtain an equivalent equation
Answer. .
Example Solve the equation.
Solution. In this case, before applying the formulas for the sum of trigonometric functions, you should use the reduction formula 
. As a result, we obtain an equivalent equation
Answer.
, 
.
Solving equations by converting the product of trigonometric functions into a sum
When solving a number of equations, formulas are used.
Example solve the equation
Solution.
Answer. , .
Example Solve the equation.
Solution. Applying the formula, we obtain an equivalent equation:
Answer. .
Solving Equations Using Reduction Formulas
When solving a wide range of trigonometric equations, formulas play a key role.
Example Solve the equation.
Solution. Applying the formula, we obtain an equivalent equation.
Answer. ; .
Solving Equations Using Triple Argument Formulas
Example Solve the equation.
Solution. We apply the formula, we get the equation
Answer. ; .
Example
solve the equation 
.
Solution. Applying the formulas for lowering the degree, we get: 
. Applying we get:
Answer. ; .
Equality of trigonometric functions of the same name
Example Solve the equation.
Solution.
Answer. , .
Example
solve the equation 
.
Solution. Let's transform the equation.
Answer. .
Example It is known that and satisfy the equation
Find the sum.
Solution. It follows from the equation that
Answer. .
Consider sums of the form
These sums can be converted to a product by multiplying and dividing them by , then we get
This technique can be used to solve some trigonometric equations, but it should be borne in mind that as a result, extraneous roots may appear. Here is a generalization of these formulas:
Example Solve the equation.
Solution. It can be seen that the set is a solution to the original equation. Therefore, multiplying the left and right sides of the equation by does not lead to the appearance of extra roots.
We have 
.
Answer. ; .
Example Solve the equation.
Solution. We multiply the left and right sides of the equation by and applying the formulas for converting the product of trigonometric functions into a sum, we obtain
This equation is equivalent to the set of two equations and , whence and .
Since the roots of the equation are not the roots of the equation, then from the resulting sets of solutions should be excluded. So in the set you need to exclude .
Answer. and , .
Example
solve the equation 
.
Solution. Let's transform the expression:
The equation will be written in the form:
Answer. .
Reduction of trigonometric equations to algebraic ones
Reducing to square
If the equation looks like
then the replacement brings it to a square, because 
() and.
If instead of the term there is , then the required replacement will be .
The equation
reduces to the quadratic equation
presentation as 
. It is easy to check that for which , are not roots of the equation, and by making the change , the equation is reduced to a quadratic one.
Example Solve the equation.
Solution. Let's move it to the left side, replace it with , and express through and .
After simplifications, we get: . Divide term by term by , make the substitution :
Returning to , we find 
.
Equations homogeneous with respect to ,
Consider an equation of the form
where , , , ..., , are real numbers. In each term on the left side of the equation, the degrees of monomials are equal, i.e., the sum of the degrees of the sine and cosine is the same and equal to. Such an equation is called homogeneous relative to and , and the number is called homogeneity indicator .
It is clear that if , then the equation will take the form:
whose solutions are the values for which , i.e., the numbers , . The second equation, written in brackets, is also homogeneous, but the degrees are 1 lower.
If , then these numbers are not the roots of the equation.
When we get: , and the left side of equation (1) takes the value .
So, for , and , therefore, both sides of the equation can be divided by . As a result, we get the equation:
which, by substitution, is easily reduced to the algebraic one:
Homogeneous equations with homogeneity index 1. At , we have the equation .
If , then this equation is equivalent to the equation , , whence , .
Example Solve the equation.
Solution. This equation is homogeneous of the first degree. Dividing both its parts by we get: , , , .
Answer. .
Example At , we obtain a homogeneous equation of the form
Solution.
If , then we divide both sides of the equation by , we get the equation 
, which can be easily reduced to a square by substitution: 
. If a 
, then the equation has real roots , . The original equation will have two groups of solutions: , , .
If a 
, then the equation has no solutions.
Example Solve the equation.
Solution. This equation is homogeneous of the second degree. Divide both sides of the equation by , we get: . Let , then , , . , , ; , , .
Answer.
.
The equation is reduced to an equation of the form
To do this, it suffices to use the identity 
In particular, the equation reduces to a homogeneous one if replaced by 
, then we get the equivalent equation:
Example Solve the equation.
Solution. Let's transform the equation to a homogeneous one:
Divide both sides of the equation by 
, we get the equation:
Let , then we come to the quadratic equation: 
, , 
, 
, .
Answer.
.
Example Solve the equation.
Solution. Let's square both sides of the equation, given that they have positive values: , ,
Let , then we get 
, , .
Answer. .
Equations Solved Using Identities 
It is useful to know the following formulas:
Example Solve the equation.
Solution. Using, we get
Answer.
We offer not the formulas themselves, but the way to derive them:
Consequently,
Likewise, .
Example
solve the equation 
.
Solution. Let's transform the expression:
The equation will be written in the form:
Taking , we get . , . Consequently
Answer. .
Universal trigonometric substitution
Trigonometric equation of the form
where --- a rational function with the help of formulas -- , as well as with the help of formulas -- can be reduced to a rational equation with respect to the arguments , , , , after which the equation can be reduced to an algebraic rational equation with respect to using the formulas of the universal trigonometric substitution
It should be noted that the use of formulas can lead to a narrowing of the ODZ of the original equation, since it is not defined at the points , so in such cases it is necessary to check whether the angles are the roots of the original equation.
Example Solve the equation.
Solution. According to the task. Applying the formulas and making the substitution , we get
whence and, therefore, .
Equations of the form
Equations of the form , where is a polynomial, are solved by changing the unknowns
Example Solve the equation.
Solution. Making the substitution and taking into account that , we get
where , . --- extraneous root, because . Equation roots 
are .
Use of Limited Functions
In the practice of centralized testing, it is not uncommon to encounter equations whose solution is based on the boundedness of the functions and . For example:
Example Solve the equation.
Solution. Since , , then the left-hand side does not exceed and is equal to , if
To find the values that satisfy both equations, we proceed as follows. We solve one of them, then among the found values we select those that satisfy the other.
Let's start with the second one: , . Then , 
.
It is clear that only for even numbers will be .
Answer. .
Another idea is realized by solving the following equation:
Example
solve the equation 
.
Solution. Let's use the property of the exponential function: , 
.
Adding these inequalities term by term, we have:
Therefore, the left side of this equation is equal if and only if the two equalities hold:
i.e. it can take the values , , , or it can take the values , .
Answer. , .
Example
solve the equation 
.
Solution., . Consequently, 
.
Answer. .
Example solve the equation
Solution. Denote , then from the definition of the inverse trigonometric function we have 
and 
.
Since , the inequality follows from the equation, i.e. . Since and , then and . However, and therefore.
If and , then . Since it was previously established that , then .
Answer. , .
Example solve the equation
Solution. The range of valid values of the equation is .
Let us first show that the function
For any, it can take only positive values.
Let's represent the function as follows: .
Since , then , i.e., 
.
Therefore, to prove the inequality , it is necessary to show that 
. To this end, we cube both parts of this inequality, then
The resulting numerical inequality indicates that . If we also take into account that , then the left side of the equation is non-negative.
Consider now the right side of the equation.
Because 
, then
However, it is known that 
. It follows from here that , i.e. the right side of the equation does not exceed . It was previously proved that the left side of the equation is non-negative, therefore equality in can only be in the case when both its parts are equal, and this is possible only for .
Answer. .
Example solve the equation
Solution. Denote and 
. Applying the Cauchy-Bunyakovsky inequality, we obtain . Hence it follows that 
. On the other hand, there is 
. Therefore, the equation has no roots.
Answer. .
Example Solve the equation:
Solution. Let's rewrite the equation in the form:
Answer. .
Functional methods for solving trigonometric and combined equations
Not every equation as a result of transformations can be reduced to an equation of one or another standard form, for which there is a certain solution method. In such cases, it turns out to be useful to use such properties of the functions and as monotonicity, boundedness, evenness, periodicity, etc. So, if one of the functions decreases, and the second increases on the interval, then if the equation has a root on this interval, this root is unique, and then, for example, it can be found by selection. If the function is bounded from above, and , and the function is bounded from below, and , then the equation is equivalent to the system of equations
Example solve the equation
Solution. We transform the original equation to the form
and solve it as a square with respect to . Then we get
Let's solve the first set equation. Taking into account the boundedness of the function , we come to the conclusion that the equation can have a root only on the interval . On this interval, the function increases, and the function 
decreases. Therefore, if this equation has a root, then it is unique. We find by selection.
Answer. .
Example solve the equation
Solution. Let , and 
, then the original equation can be written as a functional equation . Since the function is odd, then . In this case, we get the equation
Since , and is monotonic on , the equation is equivalent to the equation , i.e. 
, which has a single root .
Answer. .
Example
solve the equation 
.
Solution. Based on the theorem on the derivative of a complex function, it is clear that the function 
decreasing (function decreasing, increasing, decreasing). From this it is clear that the function 
defined on , decreasing. Therefore, this equation has at most one root. Because 
, then
Answer. .
Example Solve the equation.
Solution. Consider the equation on three intervals.
a) Let . Then on this set the original equation is equivalent to the equation . Which has no solutions on the interval, since 
, , a . On the interval, the original equation also has no roots, because 
, a .
b) Let . Then on this set the original equation is equivalent to the equation
whose roots on the interval are the numbers , , , .
c) Let . Then on this set the original equation is equivalent to the equation
Which has no solutions on the interval, since , but . The equation also has no solutions on the interval, since , , a .
Answer. , , , .
Symmetry method
The symmetry method is convenient to use when the task formulation contains the requirement that the solution of an equation, inequality, system, etc. be unique. or an exact indication of the number of solutions. In this case, any symmetry of the given expressions should be detected.
It is also necessary to take into account the variety of different possible types of symmetry.
Equally important is the strict observance of logical stages in reasoning with symmetry.
Usually, symmetry allows us to establish only the necessary conditions, and then we need to check their sufficiency.
Example Find all values of the parameter for which the equation has a unique solution.
Solution. Note that and are even functions, so the left side of the equation is an even function.
So if is a solution to an equation, then there is also a solution to the equation. If is the only solution to the equation, then necessary , .
Let's select possible values , requiring that be the root of the equation.
We immediately note that other values cannot satisfy the condition of the problem.
But it is not yet known whether all those selected actually satisfy the condition of the problem.
Adequacy.
1) , the equation will take the form 
.
2) , the equation will take the form:
Obviously, for all and 
. Therefore, the last equation is equivalent to the system:
Thus, we have proved that for , the equation has a unique solution.
Answer. .
Solution with function exploration
Example Prove that all solutions of the equation
Whole numbers.
Solution. The main period of the original equation is . Therefore, we first study this equation on the segment .
Let's transform the equation to the form:
With the help of a calculator we get:
If , then from the previous equalities we get:
Solving the resulting equation, we get: .
The performed calculations provide an opportunity to assume that the roots of the equation belonging to the interval are , and .
Direct verification confirms this hypothesis. Thus, it is proved that the roots of the equation are only integers , .
Example
Solve the Equation 
.
Solution. Find the main period of the equation. The main period of the function is . The main period of the function is . The least common multiple of the numbers and is equal to . Therefore, the main period of the equation is . Let .
Obviously, is a solution to the equation. On the interval. The function is negative. Therefore, other roots of the equation should be sought only on the intervals x and .
With the help of a microcalculator, we first find the approximate values of the roots of the equation. To do this, we compile a table of function values 
on intervals and ; i.e., on the intervals and .
| 0 | 0 | 202,5 | 0,85355342 |
| 3 | -0,00080306 | 207 | 0,6893642 |
| 6 | -0,00119426 | 210 | 0,57635189 |
| 9 | -0,00261932 | 213 | 0,4614465 |
| 12 | -0,00448897 | 216 | 0,34549155 |
| 15 | -0,00667995 | 219 | 0,22934931 |
| 18 | -0,00903692 | 222 | 0,1138931 |
| 21 | -0,01137519 | 225 | 0,00000002 |
| 24 | -0,01312438 | 228 | -0,11145712 |
| 27 | -0,01512438 | 231 | -0,21961736 |
| 30 | -0,01604446 | 234 | -0,32363903 |
| 33 | -0,01597149 | 237 | -0,42270819 |
| 36 | -0,01462203 | 240 | -0,5160445 |
| 39 | -0,01170562 | 243 | -0,60290965 |
| 42 | -0,00692866 | 246 | -0,65261345 |
| 45 | 0,00000002 | 249 | -0,75452006 |
| 48 | 0,00936458 | 252 | -0,81805397 |
| 51 | 0,02143757 | 255 | -0,87270535 |
| 54 | 0,03647455 | 258 | -0,91803444 |
| 57 | 0,0547098 | 261 | -0,95367586 |
| 60 | 0,07635185 | 264 | -0,97934187 |
| 63 | 0,10157893 | 267 | -0,99482505 |
| 66 | 0,1305352 | 270 | -1 |
| 67,5 | 0,14644661 |
The following hypotheses are easily seen from the table: the roots of the equation belonging to the segment are numbers: ; ; . Direct verification confirms this hypothesis.
Answer.
; 
; .
Solving trigonometric inequalities using the unit circle
When solving trigonometric inequalities of the form , where is one of the trigonometric functions, it is convenient to use a trigonometric circle in order to most clearly present the solution of the inequality and write down the answer. The main method for solving trigonometric inequalities is to reduce them to the simplest inequalities of the type . Let's look at an example of how to solve such inequalities.
Example Solve the inequality.
Solution. Let's draw a trigonometric circle and mark on it the points for which the ordinate is greater than .
For the solution of this inequality will be . It is also clear that if some number differs from some number from the indicated interval by , then it will also be no less than . Therefore, to the ends of the found segment of the solution, you just need to add . Finally, we get that the solutions of the original inequality will be all 
.
Answer.
.
To solve inequalities with tangent and cotangent, the concept of a line of tangents and cotangents is useful. These are the lines and, respectively (in the figure (1) and (2)), touching the trigonometric circle.
It is easy to see that if you build a ray with origin at the origin, making an angle with the positive direction of the abscissa axis, then the length of the segment from the point to the point of intersection of this ray with the line of tangents is exactly equal to the tangent of the angle that this ray makes with the abscissa axis. A similar observation holds for the cotangent.
Example Solve the inequality.
Solution. Denote , then the inequality will take the form of the simplest: . Consider an interval with a length equal to the least positive period (LPP) of the tangent. On this segment, using the line of tangents, we establish that . We now recall what needs to be added, since the RPE of the function . So, 
. Returning to the variable , we get that .
Answer.
.
It is convenient to solve inequalities with inverse trigonometric functions using graphs of inverse trigonometric functions. Let's show how this is done with an example.
Solving trigonometric inequalities by a graphical method
Note that if --- is a periodic function, then to solve the inequality, it is necessary to find its solutions on a segment whose length is equal to the period of the function . All solutions of the original inequality will consist of the values found, as well as all that differ from those found by any integer number of periods of the function.
Consider the solution of the inequality ().
Since , then the inequality has no solutions for . If , then the set of solutions to the inequality is the set of all real numbers.
Let . The sine function has the smallest positive period, so the inequality can be solved first on a segment of length , for example, on a segment. We build graphs of functions and (). are given by inequalities of the form: and, whence,
In this paper, methods for solving trigonometric equations and inequalities, both the simplest and the Olympiad level, were considered. The main methods for solving trigonometric equations and inequalities were considered, both specific --- characteristic only for trigonometric equations and inequalities --- and general functional methods for solving equations and inequalities, as applied to trigonometric equations.
The thesis provides basic theoretical information: the definition and properties of trigonometric and inverse trigonometric functions; expression of trigonometric functions in terms of other trigonometric functions, which is very important for converting trigonometric expressions, especially those containing inverse trigonometric functions; in addition to the basic trigonometric formulas, well known from the school course, formulas are given that simplify expressions containing inverse trigonometric functions. The solution of elementary trigonometric equations, the method of factoring, methods of reducing trigonometric equations to algebraic ones are considered. In view of the fact that the solutions of trigonometric equations can be written in several ways, and the form of these solutions does not allow one to immediately determine whether these solutions are the same or different, a general scheme for solving trigonometric equations is considered and the transformation of groups of general solutions of trigonometric equations is considered in detail. Methods for solving elementary trigonometric inequalities, both on a unit circle and by a graphical method, are considered in detail. The process of solving non-elementary trigonometric inequalities through elementary inequalities and the method of intervals already well known to schoolchildren are described. The solutions of typical tasks for the selection of roots are given. The necessary theoretical information for the selection of roots is given: the partition of the set of integers into non-intersecting subsets, the solution of equations in integers (diophantine).
The results of this thesis work can be used as educational material in the preparation of term papers and theses, in the preparation of electives for schoolchildren, as well as the work can be used in preparing students for entrance exams and centralized testing.
Vygodsky Ya.Ya., Handbook of elementary mathematics. /Vygodsky Ya.Ya. --- M.: Nauka, 1970.
Igudisman O., Mathematics at the oral exam / Igudisman O. --- M .: Iris press, Rolf, 2001.
Azarov A.I., equations / Azarov A.I., Gladun O.M., Fedosenko V.S. --- Minsk: Trivium, 1994.
Litvinenko V.N., Workshop on elementary mathematics / Litvinenko V.N. --- M .: Education, 1991.
Sharygin I.F., Optional course in mathematics: problem solving / Sharygin I.F., Golubev V.I. --- M.: Enlightenment, 1991.
Bardushkin V., Trigonometric equations. Selection of roots / V. Bardushkin, A. Prokofiev.// Mathematics, No. 12, 2005 p. 23--27.
Vasilevsky A.B., Assignments for extracurricular work in mathematics / Vasilevsky A.B. --- Mn.: People's Asveta. 1988. --- 176s.
Sapunov P. I., Transformation and union of groups of general solutions of trigonometric equations / Sapunov P. I. // Mathematical education, issue No. 3, 1935.
Borodin P., Trigonometry. Materials of entrance examinations at Moscow State University [text] / P. Borodin, V. Galkin, V. Panferov, I. Sergeev, V. Tarasov // Mathematics No. 1, 2005 p. 36--48.
Samusenko A.V., Mathematics: Typical mistakes of applicants: Reference manual / Samusenko A.V., Kazachenok V.V. --- Minsk: Higher School, 1991.
Azarov A.I., Functional and graphical methods for solving examination problems / Azarov A.I., Barvenov S.A., --- Minsk: Aversev, 2004.
Solution of the simplest trigonometric equations
First, let's recall the formulas for solving the simplest trigonometric equations.
- $sinx=a$
- $cosx=a$
- $tgx=a$
- $ctgx=a$
Solution of the simplest trigonometric inequalities.
To solve the simplest trigonometric inequalities, we first need to solve the corresponding equation, and then, using the trigonometric circle, find a solution to the inequality. Consider the solutions of the simplest trigonometric inequalities by examples.
Example 1
$sinx\ge \frac(1)(2)$
Find a solution to the trigonometric inequality $sinx=\frac(1)(2)$
\ \
Figure 1. Solution of the inequality $sinx\ge \frac(1)(2)$.
Since the inequality has a “greater than or equal” sign, the solution lies on the upper arc of the circle (with respect to the solution of the equation).
Answer: $\left[\frac(\pi )(6)+2\pi n,\frac(5\pi )(6)+2\pi n\right]$.
Example 2
Find a solution to the trigonometric inequality $cosx=\frac(\sqrt(3))(2)$
\ \
Note the solution on the trigonometric circle
Since the inequality has a “less than” sign, the solution lies on the arc of the circle located to the left (with respect to the solution of the equation).
Answer: $\left(\frac(\pi )(6)+2\pi n,\frac(11\pi )(6)+2\pi n\right)$.
Example 3
$tgx\le \frac(\sqrt(3))(3)$
Find a solution to the trigonometric inequality $tgx=\frac(\sqrt(3))(3)$
\ \
Here we also need a domain of definition. As we remember, the tangent function $x\ne \frac(\pi )(2)+\pi n,n\in Z$
Note the solution on the trigonometric circle
Figure 3. Solution of the inequality $tgx\le \frac(\sqrt(3))(3)$.
Since the inequality has a “less than or equal to” sign, the solution lies on the arcs of the circle marked in blue in Figure 3.
Answer: $\ \left(-\frac(\pi )(2)+2\pi n\right.,\left.\frac(\pi )(6)+2\pi n\right]\cup \left (\frac(\pi )(2)+2\pi n,\right.\left.\frac(7\pi )(6)+2\pi n\right]$
Example 4
Find a solution to the trigonometric inequality $ctgx=\sqrt(3)$
\ \
Here we also need a domain of definition. As we remember, the tangent function $x\ne \pi n,n\in Z$
Note the solution on the trigonometric circle
Figure 4. Solution to the inequality $ctgx\le \sqrt(3)$.
Since the inequality has a “greater than” sign, the solution lies on the arcs of the circle marked in blue in Figure 4.
Answer: $\ \left(2\pi n,\frac(\pi )(6)+2\pi n\right)\cup \left(\pi +2\pi n,\frac(7\pi )( 6)+2\pi n\right)$
DEFINITION
Trigonometric inequalities are inequalities that contain a variable under the sign of a trigonometric function.
Solving trigonometric inequalities
The solution of trigonometric inequalities often comes down to solving the simplest trigonometric inequalities of the form: \(\ \sin x a \), \(\ \cos x > a \), \(\ \operatorname(tg) x > a \), \(\ \ operatorname(ctg) x > a \), \(\ \sin x \leq a \), \(\ \cos x \leq a \), \(\ \operatorname(tg) x \leq a \), \ (\ \operatorname(ctg) x \leq a \), \(\ \sin x \geq a \), \(\ \cos \geq a \), \(\ \operatorname(tg) x \geq a \ ), \(\ \operatorname(tg) x \geq a \)
The simplest trigonometric inequalities are solved graphically or using a unit trigonometric circle.
By definition, the sine of the angle \(\ \alpha \) is the ordinate of the point \(\ P_(\alpha)(x, y) \) of the unit circle (Fig. 1), and the cosine is the abscissa of this point. This fact is used in solving the simplest trigonometric inequalities with cosine and sine using the unit circle.
Examples of solving trigonometric inequalities
Solve the inequality \(\ \sin x \leq \frac(\sqrt(3))(2) \)
Since \(\ \left|\frac(\sqrt(3))(2)\right| , this inequality has a solution and can be solved in two ways
First way. Let's solve this inequality graphically. To do this, we construct in the same coordinate system a graph of the sine \(\ y=\sin x \) (Fig. 2) and the straight line \(\ y=\frac(\sqrt(3))(2) \)
Let's select the intervals where the sinusoid is located below the graph of the straight line \(\ y=\frac(\sqrt(3))(2) \) . Find the abscissas \(\ x_(1) \) and \(\ x_(2) \) of the intersection points of these graphs: \(\ x_(1)=\pi-\arcsin \frac(\sqrt(3))(2 )=\pi-\frac(\pi)(3)=\frac(2 \pi)(3) x_(2)=\arcsin \frac(\sqrt(3))(2)+2 \pi=\ frac(\pi)(3)+2 \pi=\frac(7 \pi)(3) \)
We got the interval \(\ \left[-\frac(4 \pi)(3) ; \frac(\pi)(3)\right] \) but since the function \(\ y=\sin x \) is periodic and has a period \(\ 2 \pi \) , then the answer is the union of intervals: \(\ \left[\frac(2 \pi)(3)+2 \pi k ; \frac(7 \pi)(3)+ 2 \pi k\right] \), \(\ k \in Z \)
The second way. Construct a unit circle and a line \(\ y=\frac(\sqrt(3))(2) \) , denote their intersection points \(\ P_(x_(1)) \) and \(\ P_(x_(2 )) \) (Fig. 3). The solution to the original inequality will be the set of ordinate points that are less than \(\ \frac(\sqrt(3))(2) \) . Let's find the value of \(\ \boldsymbol(I)_(1) \) and \(\ \boldsymbol(I)_(2) \) by going counterclockwise, \(\ x_(1) Fig. 3
\(\ x_(1)=\pi-\arcsin \frac(\sqrt(3))(2)=\pi-\frac(\pi)(3)=\frac(2 \pi)(3) x_ (2)=\arcsin \frac(\sqrt(3))(2)+2 \pi=\frac(\pi)(3)+2 \pi=\frac(7 \pi)(3) \)
Taking into account the periodicity of the sine function, we finally obtain the intervals \(\ \left[\frac(2 \pi)(3)+2 \pi k ; \frac(7 \pi)(3)+2 \pi\right] \), \(\k\in Z\)
Solve the inequality \(\ \sin x>2 \)
The sine is a bounded function: \(\ |\sin x| \leq 1 \) , and the right side of this inequality is greater than one, so there are no solutions.
Solve the inequality \(\ \cos x>\frac(1)(2) \)
This inequality can be solved in two ways: graphically and using a unit circle. Let's consider each of the methods.
First way. Let's depict in one coordinate system the functions that describe the left and right parts of the inequality, that is, \(\ y=\cos x \) and \(\ y=\frac(1)(2) \) . Let us select the intervals where the graph of the cosine function \(\ y=\cos x \) is located above the graph of the straight line \(\ y=\frac(1)(2) \) (Fig. 4).
Find the abscissas of the points \(\ \boldsymbol(x)_(1) \) and \(\ x_(2) \) - the points of intersection of the graphs of the functions \(\ y=\cos x \) and \(\ y=\frac (1)(2) \) , which are the ends of one of the intervals on which the indicated inequality holds. \(\ x_(1)=-\arccos \frac(1)(2)=-\frac(\pi)(3) \); \(\ x_(1)=\arccos \frac(1)(2)=\frac(\pi)(3) \)
Considering that the cosine is a periodic function, with a period \(\ 2 \pi \) , the answer is the value \(\ x \) from the intervals \(\ \left(-\frac(\pi)(3)+2 \pi k ; \frac(\pi)(3)+2 \pi k\right) \), \(\ k \in Z \)
The second way. Let's construct a unit circle and a straight line \(\ x=\frac(1)(2) \) (since the x-axis corresponds to the cosines on the unit circle). Let \(\ P_(x_(1)) \) and \(\ P_(x_(2)) \) (Fig. 5) be the intersection points of the line and the unit circle. The solution to the original equation will be the set of abscissa points that are less than \(\ \frac(1)(2) \) . Find the value of \(\ x_(1) \) and \(\ 2 \) , making a counterclockwise tour so that \(\ x_(1) Taking into account the periodicity of the cosine, we finally obtain the intervals \(\ \left(-\frac (\pi)(3)+2 \pi k ;\frac(\pi)(3)+2 \pi k\right) \),\(\ k \in Z \)
Solve the inequality \(\ \operatorname(ctg) x \leq-\frac(\sqrt(3))(3) \)
Let's plot graphs of functions \(\ y=\operatorname(ctg) x \), \(\ y=-\frac(\sqrt(3))(3) \) in one coordinate system
Let's select the intervals where the graph of the function \(\ y=\operatorname(ctg) x \) is not higher than the graph of the straight line \(\ y=-\frac(\sqrt(3))(3) \) (Fig. 6) .
Find the abscissa of the point \(\ x_(0) \) , which is the end of one of the intervals on which the inequality \(\ x_(0)=\operatorname(arcctg)\left(-\frac(\sqrt(3))( 3)\right)=\pi-\operatorname(arcctg)\left(\frac(\sqrt(3))(3)\right)=\pi-\frac(\pi)(3)=\frac(2 \pi)(3) \)
The other end of this gap is the point \(\ \pi \) , and the function \(\ y=\operatorname(ctg) x \) is undefined at this point. Thus, one of the solutions to this inequality is the interval \(\ \frac(2 \pi)(3) \leq x
Trigonometric inequalities with complex argument
Trigonometric inequalities with a complex argument can be reduced to the simplest trigonometric inequalities using a substitution. After solving it, the reverse substitution is made and the original unknown is expressed.
Solve the inequality \(\ 2 \cos \left(2 x+100^(\circ)\right) \leq-1 \)
Express the cosine on the right side of this inequality: \(\ \cos \left(2 x+100^(\circ)\right) \leq-\frac(1)(2) \)
We carry out the replacement \(\ t=2 x+100^(\circ) \) , after which this inequality is transformed to the simplest inequality \(\ \cos t \leq-\frac(1)(2) \)
Let's solve it using the unit circle. Let's construct a unit circle and a line \(\ x=-\frac(1)(2) \) . Let us denote \(\ P_(1) \) and \(\ P_(2) \) as the points of intersection of the line and the unit circle (Fig. 7).
The solution to the original inequality will be the set of abscissa points, which are at most \(\ -\frac(1)(2) \). The point \(\ P_(1) \) corresponds to the angle \(\ 120^(\circ) \) , and the point \(\ P_(2) \) . Thus, given the cosine period, we get \(\ 120^(\circ)+360^(\circ) \cdot n \leq t \leq 240^(\circ)+360^(\circ) \cdot n \) , \(\ n \in Z \)
We make the reverse substitution \(\ t=2 x+100^(\circ) 120^(\circ)+360^(\circ) \cdot n \leq 2 x+100^(\circ) \leq 240^(\ circ)+360^(\circ) \cdot n \), \(\ n \in Z \)
We express \(\ \mathbf(x) \), to do this, first subtract \(\ 100^(\circ) 120^(\circ)-100^(\circ)+360^(\circ) \ cdot n \leq 2 x+100^(\circ)-100^(\circ) \leq 240^(\circ)-100^(\circ)+360^(\circ) \cdot n \), \( \n\in Z\); \(\ 20^(\circ)+360^(\circ) \cdot n \leq 2 x \leq 140^(\circ)+360^(\circ) \cdot n \), \(\ n \in Z\)
and then, divide by 2 \(\ \frac(20^(\circ)+360^(\circ) \cdot n)(2) \leq \frac(2 x)(2) \leq \frac(140^ (\circ)+360^(\circ) \cdot n)(2) \), \(\ n \in Z \); \(\ 10^(\circ)+180^(\circ) \cdot n \leq x \leq 70^(\circ)+180^(\circ) \cdot n \), \(\ n \in Z \)
Double trigonometric inequalities
Solve the double trigonometric inequality \(\ \frac(1)(2)
Let us introduce the replacement \(\ t=\frac(x)(2) \) , then the original inequality will take the form \(\ \frac(1)(2)
Let's solve it using the unit circle. Since the ordinate axis corresponds to the sine on the unit circle, we select on it the set of ordinates of which is greater than \(\ x=\frac(1)(2) \) and less than or equal to \(\ \frac(\sqrt(2))(2 ) \) . In Figure 8, these points will be located on the arcs \(\ P_(t_(1)) \), \(\ P_(t_(2)) \) and \(\ P_(t_(3)) \), \( \ P_(t_(4)) \) . Let's find the value \(\ t_(1) \), \(\ t_(2) \), \(\ t_(3) \), \(\ t_(4) \) , making a counterclockwise tour, and \ (\ t_(1) \(\ t_(3)=\pi-\arcsin \frac(\sqrt(2))(2)=\pi-\frac(\pi)(4)=\frac(3 \ pi)(4) \); \(\ t_(4)=\pi-\arcsin \frac(1)(2)=\pi-\frac(\pi)(6)=\frac(5 \pi) (6)\)
Thus, we obtain two intervals, which, taking into account the periodicity of the sine function, can be written as follows \(\ \frac(\pi)(6)+2 \pi k \leq t \frac(\pi)(4)+2 \ pi k \quad \frac(3 \pi)(4)+2 \pi k leq \frac(x)(2) \frac(\pi)(4)+2 \pi k \), \(\ \frac(3 \pi)(4)+2 \pi k Express \(\ \mathbf( x) \), for this we multiply all sides of both inequalities by 2, we get \(\ \frac(\pi)(3)+4 \pi k \leq x
METHODS FOR SOLVING TRIGONOMETRIC INEQUALITIES
Relevance. Historically, trigonometric equations and inequalities have been given a special place in the school curriculum. We can say that trigonometry is one of the most important sections of the school course and of all mathematical science in general.
Trigonometric equations and inequalities occupy one of the central places in a high school mathematics course, both in terms of the content of the educational material and the methods of educational and cognitive activity that can and should be formed during their study and applied to solving a large number of problems of a theoretical and applied nature. .
The solution of trigonometric equations and inequalities creates the prerequisites for systematizing students' knowledge related to all educational material in trigonometry (for example, the properties of trigonometric functions, methods for transforming trigonometric expressions, etc.) and makes it possible to establish effective connections with the studied material in algebra (equations, equivalence of equations, inequalities, identical transformations of algebraic expressions, etc.).
In other words, the consideration of methods for solving trigonometric equations and inequalities involves a kind of transfer of these skills to a new content.
The significance of the theory and its numerous applications are proof of the relevance of the chosen topic. This, in turn, allows you to determine the goals, objectives and subject of research of the course work.
Purpose of the study: generalize the available types of trigonometric inequalities, basic and special methods for their solution, select a set of tasks for solving trigonometric inequalities by schoolchildren.
Research objectives:
1. Based on the analysis of the available literature on the research topic, systematize the material.
2. Give a set of tasks necessary to consolidate the topic "Trigonometric inequalities."
Object of study are trigonometric inequalities in the school mathematics course.
Subject of study: types of trigonometric inequalities and methods for their solution.
Theoretical significance is to organize the material.
Practical significance: application of theoretical knowledge in solving problems; analysis of the main frequently encountered methods for solving trigonometric inequalities.
Research methods : analysis of scientific literature, synthesis and generalization of the acquired knowledge, analysis of problem solving, search for optimal methods for solving inequalities.
§one. Types of trigonometric inequalities and basic methods for their solution
1.1. The simplest trigonometric inequalities
Two trigonometric expressions connected by a sign or > are called trigonometric inequalities.
To solve a trigonometric inequality means to find a set of values of the unknowns included in the inequality, under which the inequality is satisfied.
The main part of trigonometric inequalities is solved by reducing them to solving the simplest ones:
This may be a method of factorization, change of variable ( 
, 
etc.), where the usual inequality is first solved, and then the inequality of the form 
etc., or other ways.
The simplest inequalities are solved in two ways: using the unit circle or graphically.
Letf(x
is one of the basic trigonometric functions. To solve the inequality 
it suffices to find its solution on one period, i.e. on any segment whose length is equal to the period of the functionf
x
. Then the solution of the original inequality will be all foundx
, as well as those values that differ from those found by any integer number of periods of the function. In this case, it is convenient to use the graphical method.
Let us give an example of an algorithm for solving inequalities 
(
) and 
.
Algorithm for solving the inequality (
).
1. Formulate the definition of the sine of a numberx on the unit circle.
3. On the y-axis, mark a point with the coordinatea .
4. Through this point, draw a line parallel to the OX axis, and mark the points of intersection of it with the circle.
5. Select an arc of a circle, all points of which have an ordinate less thana .
6. Specify the direction of the bypass (counterclockwise) and write down the answer by adding the period of the function to the ends of the interval2πn
,
.
Algorithm for solving the inequality .
1. Formulate the definition of the tangent of a numberx on the unit circle.
2. Draw a unit circle.
3. Draw a line of tangents and mark a point on it with an ordinatea .
4. Connect this point to the origin and mark the point of intersection of the resulting segment with the unit circle.
5. Select an arc of a circle, all points of which have an ordinate on the tangent line that is less thana .
6. Indicate the direction of the traversal and write down the answer, taking into account the scope of the function, adding a periodpn
,
(the number on the left side of the record is always less than the number on the right side).
Graphical interpretation of solutions to the simplest equations and formulas for solving inequalities in a general form are given in the appendix (Appendices 1 and 2).
Example 1
Solve the inequality 
.
Draw a line on the unit circle 
, which intersects the circle at points A and B.
All valuesy
on the interval NM more
, all points of the arc AMB satisfy this inequality. At all angles of rotation, large 
, but smaller 
,
will take on values greater than
(but not more than one).
Fig.1
Thus, the solution of the inequality will be all values in the interval 
, i.e. 
. In order to get all solutions of this inequality, it is enough to add to the ends of this interval 
, where 
, i.e. 
,
.
Note that the values 
and 
are the roots of the equation 
,
those. 
;
.
Answer: 
, .
1.2. Graphic method
In practice, a graphical method for solving trigonometric inequalities is often useful. Consider the essence of the method on the example of the inequality 
:
1. If the argument is complex (different fromX ), then we replace it witht .
2. We build in one coordinate planetoOy
function graphs 
and 
.
3. We find suchtwo adjacent points of intersection of graphs, between whichsinusoidsituatedabove
straight . Find the abscissas of these points.
4. Write a double inequality for the argumentt , considering the cosine period (t will be between the found abscissas).
5. Do a reverse substitution (return to the original argument) and express the valueX from a double inequality, we write the answer as a numerical interval.
Example 2 Solve the inequality: .
When solving inequalities by a graphical method, it is necessary to build graphs of functions as accurately as possible. Let's transform the inequality to the form:
Let us construct graphs of functions in one coordinate system 
and 
(Fig. 2).
Fig.2
Function graphs intersect at a pointBUT
with coordinates 
; 
. In between 
graph points 
below the chart points 
. And when function values are the same. That's why at 
.
Answer: 
.
1.3. Algebraic method
Quite often, the original trigonometric inequality, by a well-chosen substitution, can be reduced to an algebraic (rational or irrational) inequality. This method involves transforming the inequality, introducing a substitution, or replacing a variable.
Let's consider the application of this method on specific examples.
Example 3
Reduction to the simplest form 
.
(Fig. 3)
Fig.3
, 
.
Answer: 
,
Example 4 Solve the inequality:
ODZ: 
, 
.
Using formulas: 
, 
we write the inequality in the form: 
.
Or, assuming 
after simple transformations we get
,
,
.
Solving the last inequality by the interval method, we obtain:
Fig.4
, respectively 
. Then from Fig. 4 follows 
, where 
.
Fig.5
Answer: 
, 
.
1.4. Spacing Method
The general scheme for solving trigonometric inequalities by the interval method:
Using trigonometric formulas, factorize.
Find breakpoints and zeros of the function, put them on the circle.
Take any pointTo (but not found earlier) and find out the sign of the product. If the product is positive, then put a point outside the unit circle on the ray corresponding to the angle. Otherwise, put the point inside the circle.
If a point occurs an even number of times, we call it a point of even multiplicity; if an odd number of times, we call it a point of odd multiplicity. Draw arcs as follows: start from a pointTo , if the next point is of odd multiplicity, then the arc intersects the circle at this point, but if the point is of even multiplicity, then it does not intersect.
Arcs behind a circle are positive gaps; inside the circle are negative intervals.
Example 5 Solve the inequality
, 
.
Points of the first series: 
.
Points of the second series: 
.
Each point occurs an odd number of times, that is, all points of odd multiplicity.
Find out the sign of the product at 
: . We mark all points on the unit circle (Fig. 6):
Rice. 6
Answer: 
, 
; 
, 
; 
, 
.
Example 6 . Solve the inequality.
Solution:
Let's find the zeros of the expression .
Getaem :
,
;
, 
;
, 
;
, 
;
On the unit circle, series valuesX
1
represented by dots 
. SeriesX
2
gives points 
. A seriesX
3
we get two points 
. Finally, a seriesX
4
will represent points 
. We put all these points on the unit circle, indicating in parentheses next to each of its multiplicity.
Now let the number 
will be equal. We make an estimate by the sign:
So the pointA
should be chosen on the beam forming the angle 
with beamOh,
outside the unit circle. (Note that the auxiliary beamO
A
it doesn't have to be shown in the picture. DotA
selected approximately.)
Now from the pointA
we draw a wavy continuous line sequentially to all the marked points. And at the points our line passes from one region to another: if it was outside the unit circle, then it passes into it. Approaching the point 
, the line returns to the inner region, since the multiplicity of this point is even. Similarly at the point 
(with an even multiplicity) the line has to be rotated to the outer region. So, we drew a certain picture depicted in Fig. 7. It helps to highlight the desired areas on the unit circle. They are marked with a "+".
Fig.7
Final answer:
Note. If the wavy line, after traversing all the points marked on the unit circle, cannot be returned to the pointA , without crossing the circle in an “illegal” place, this means that an error was made in the solution, namely, an odd number of roots were omitted.
Answer: .
§2. A set of tasks for solving trigonometric inequalities
In the process of developing the ability of schoolchildren to solve trigonometric inequalities, 3 stages can also be distinguished.
1. preparatory,
2. formation of skills to solve the simplest trigonometric inequalities;
3. introduction of trigonometric inequalities of other types.
The purpose of the preparatory stage is that it is necessary to form in schoolchildren the ability to use a trigonometric circle or graph to solve inequalities, namely:
Ability to solve simple inequalities of the form ,
, ,
,
using the properties of the sine and cosine functions;
Ability to make double inequalities for arcs of a numerical circle or for arcs of graphs of functions;
Ability to perform various transformations of trigonometric expressions.
It is recommended to implement this stage in the process of systematizing schoolchildren's knowledge about the properties of trigonometric functions. The main means can be tasks offered to students and performed either under the guidance of a teacher or independently, as well as skills gained in solving trigonometric equations.
Here are examples of such tasks:
1
. Mark a point on the unit circle 
, if
.
2.
In what quarter of the coordinate plane is the point , if 
equals: 
3.
Mark points on the trigonometric circle , if:
4. Bring the expression to trigonometric functionsIquarters.
a) 
,
b) 
,
in) 
5. Given the arc MR.M - middleIth quarter,R - middleIIth quarter. Restrict the value of a variablet for: (compose a double inequality) a) arc MP; b) RM arcs.
6. Write a double inequality for the selected sections of the graph:
Rice. one
7.
Solve inequalities 
, 
, 
, 
.
8. Convert expression .
At the second stage of learning to solve trigonometric inequalities, we can offer the following recommendations related to the methodology for organizing students' activities. At the same time, it is necessary to focus on the students' skills to work with a trigonometric circle or a graph, which are formed during the solution of the simplest trigonometric equations.
First, it is possible to motivate the expediency of obtaining a general method for solving the simplest trigonometric inequalities by referring, for example, to an inequality of the form 
.
Using the knowledge and skills acquired at the preparatory stage, students will bring the proposed inequality to the form 
, but may find it difficult to find a set of solutions to the resulting inequality, since it is impossible to solve it only using the properties of the sine function. This difficulty can be avoided by referring to the appropriate illustration (the solution of the equation graphically or using the unit circle).
Secondly, the teacher should draw students' attention to different ways of completing the task, give an appropriate example of solving the inequality both graphically and using a trigonometric circle.
Consider such options for solving the inequality .
1. Solving the inequality using the unit circle.
In the first lesson on solving trigonometric inequalities, we will offer students a detailed solution algorithm, which in a step-by-step presentation reflects all the basic skills necessary to solve the inequality.
Step 1.Draw a unit circle, mark a point on the y-axis 
and draw a straight line through it parallel to the x-axis. This line will intersect the unit circle at two points. Each of these points depicts numbers whose sine is equal to .
Step 2This straight line divided the circle into two arcs. Let's single out the one on which numbers are displayed that have a sine greater than . Naturally, this arc is located above the drawn straight line.
Rice. 2
Step 3Let's choose one of the ends of the marked arc. Let's write down one of the numbers that is represented by this point of the unit circle 
.
Step 4In order to choose a number corresponding to the second end of the selected arc, we "pass" along this arc from the named end to the other. At the same time, we recall that when moving counterclockwise, the numbers that we will pass increase (when moving in the opposite direction, the numbers would decrease). Let's write down the number that is depicted on the unit circle by the second end of the marked arc 
.
Thus, we see that the inequality satisfy the numbers for which the inequality 
. We solved the inequality for numbers located on the same period of the sine function. Therefore, all solutions of the inequality can be written as 
Students should be asked to carefully consider the figure and figure out why all the solutions to the inequality can be written in the form 
, 
.
Rice. 3
It is necessary to draw the attention of students to the fact that when solving inequalities for the cosine function, we draw a straight line parallel to the y-axis.
Graphical way to solve the inequality.
Building charts 
and 
, given that 
.
Rice. four
Then we write the equation 
and his solution 
, 
, 
, found using formulas 
, 
, 
.
(Givingn
values 0, 1, 2, we find three roots of the composed equation). Values 
are three consecutive abscissas of the intersection points of the graphs and . Obviously, always on the interval 
the inequality 
, and on the interval 
- inequality 
. We are interested in the first case, and then adding to the ends of this interval a number that is a multiple of the sine period, we obtain a solution to the inequality as: 
, .
Rice. 5
Summarize. To solve the inequality , you need to write the corresponding equation and solve it. From the resulting formula find the roots 
and 
, and write the answer of the inequality in the form: , .
Thirdly, the fact about the set of roots of the corresponding trigonometric inequality is very clearly confirmed when solving it graphically.
Rice. 6
It is necessary to demonstrate to students that the coil, which is the solution to the inequality, repeats through the same interval, equal to the period of the trigonometric function. You can also consider a similar illustration for the graph of the sine function.
Fourthly, it is advisable to carry out work on updating students' methods of converting the sum (difference) of trigonometric functions into a product, to draw the attention of schoolchildren to the role of these techniques in solving trigonometric inequalities.
Such work can be organized through the students' independent fulfillment of the tasks proposed by the teacher, among which we highlight the following:
Fifth, students must be required to illustrate the solution of each simple trigonometric inequality using a graph or a trigonometric circle. Be sure to pay attention to its expediency, especially to the use of a circle, since when solving trigonometric inequalities, the corresponding illustration serves as a very convenient means of fixing the set of solutions to a given inequality.
Acquaintance of students with methods for solving trigonometric inequalities, which are not the simplest, should be carried out according to the following scheme: referring to a specific trigonometric inequality referring to the corresponding trigonometric equation joint search (teacher - students) for a solution independent transfer of the found technique to other inequalities of the same type.
In order to systematize students' knowledge of trigonometry, we recommend specifically selecting such inequalities, the solution of which requires various transformations that can be implemented in the process of solving it, focusing students' attention on their features.
As such productive inequalities, we can propose, for example, the following:
In conclusion, we give an example of a set of problems for solving trigonometric inequalities.
1. Solve the inequalities:
2. Solve the inequalities: 3. Find all solutions of inequalities: 4. Find all solutions of inequalities:a) 
, satisfying the condition 
;
b) 
, satisfying the condition 
.
5. Find all solutions of inequalities:
a) ;
b) ;
in) 
;
G) 
;
e) 
.
6. Solve the inequalities:
a) ;
b) ;
in) ;
G) 
;
e) ;
e) ;
and) 
.
7. Solve the inequalities:
a) 
;
b) ;
in) ;
G) .
8. Solve the inequalities:
a) ;
b) ;
in) ;
G) 
;
e) 
;
e) ;
and) 
;
h) .
It is advisable to offer tasks 6 and 7 to students studying mathematics at an advanced level, task 8 - to students in classes with in-depth study of mathematics.
§3. Special methods for solving trigonometric inequalities
Special methods for solving trigonometric equations - that is, those methods that can only be used to solve trigonometric equations. These methods are based on the use of the properties of trigonometric functions, as well as on the use of various trigonometric formulas and identities.
3.1. Sector Method
Consider the sector method for solving trigonometric inequalities. Solution of inequalities of the form 
, whereP
(
x
)
andQ
(
x
)
- rational trigonometric functions (sines, cosines, tangents and cotangents enter them rationally), similarly to the solution of rational inequalities. It is convenient to solve rational inequalities by the method of intervals on the real axis. Its analogue in solving rational trigonometric inequalities is the method of sectors in a trigonometric circle, forsinx
andcosx
(
) or a trigonometric semicircle fortgx
andctgx
(
).
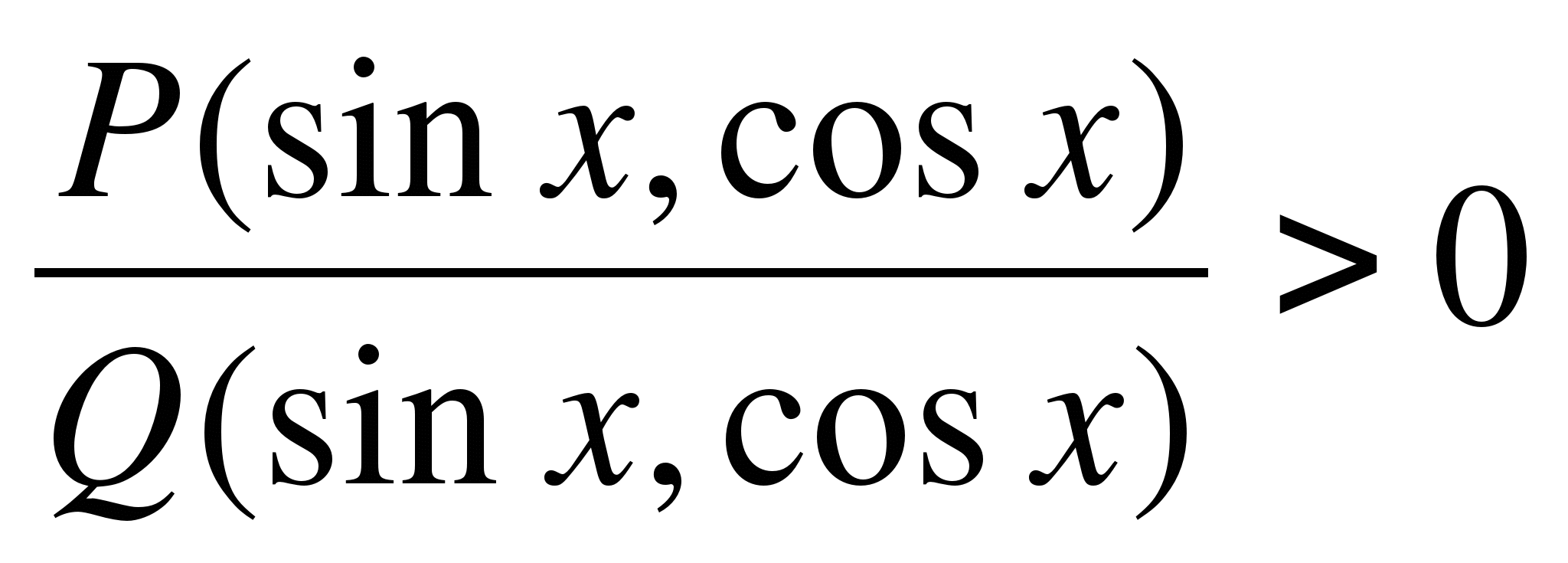
In the interval method, each linear factor of the numerator and denominator of the form 
point on the number axis 
, and when passing through this point changes sign. In the sector method, each multiplier of the form 
, where 
- one of the functionssinx
orcosx
and 
, in a trigonometric circle there correspond two angles 
and 
, which divide the circle into two sectors. When passing through and function changes sign.
The following must be remembered:
a) Multipliers of the form 
and 
, where 
, retain sign for all values 
. Such multipliers of the numerator and denominator are discarded, changing (if 
) for each such rejection, the inequality sign is reversed.
b) Multipliers of the form 
and 
are also discarded. Moreover, if these are factors of the denominator, then inequalities of the form are added to the equivalent system of inequalities 
and 
. If these are factors of the numerator, then in the equivalent system of constraints they correspond to the inequalities and in the case of strict initial inequality, and equality 
and 
in the case of a non-strict initial inequality. When dropping the multiplier 
or 
the inequality sign is reversed.
Example 1
Solve inequalities: a) 
, b) 
.
we have a function, b). Solve the inequality We have
3.2. Concentric circle method
This method is analogous to the method of parallel numerical axes in solving systems of rational inequalities.
Consider an example of a system of inequalities.
Example 5
Solve a system of simple trigonometric inequalities 
First, we solve each inequality separately (Figure 5). In the upper right corner of the figure, we will indicate for which argument the trigonometric circle is considered.
Fig.5
Next, we build a system of concentric circles for the argumentX . We draw a circle and shade it according to the solution of the first inequality, then we draw a circle of a larger radius and shade it according to the solution of the second one, then we build a circle for the third inequality and a base circle. We draw rays from the center of the system through the ends of the arcs so that they intersect all circles. We form a solution on the base circle (Figure 6).
Fig.6
Answer:
, 
.
Conclusion
All objectives of the coursework were completed. The theoretical material is systematized: the main types of trigonometric inequalities and the main methods for their solution (graphical, algebraic, method of intervals, sectors and the method of concentric circles) are given. For each method, an example of solving an inequality was given. The theoretical part was followed by the practical part. It contains a set of tasks for solving trigonometric inequalities.
This coursework can be used by students for independent work. Students can check the level of assimilation of this topic, practice in performing tasks of varying complexity.
Having worked through the relevant literature on this issue, obviously, we can conclude that the ability and skills to solve trigonometric inequalities in the school course of algebra and the beginning of analysis are very important, the development of which requires considerable effort on the part of the mathematics teacher.
Therefore, this work will be useful for teachers of mathematics, as it makes it possible to effectively organize the training of students on the topic "Trigonometric inequalities".
The study can be continued by expanding it to the final qualifying work.
List of used literature
Bogomolov, N.V. Collection of problems in mathematics [Text] / N.V. Bogomolov. – M.: Bustard, 2009. – 206 p.
Vygodsky, M.Ya. Handbook of elementary mathematics [Text] / M.Ya. Vygodsky. – M.: Bustard, 2006. – 509 p.
Zhurbenko, L.N. Mathematics in examples and tasks [Text] / L.N. Zhurbenko. – M.: Infra-M, 2009. – 373 p.
Ivanov, O.A. Elementary mathematics for schoolchildren, students and teachers [Text] / O.A. Ivanov. – M.: MTsNMO, 2009. – 384 p.
Karp, A.P. Tasks in algebra and the beginnings of analysis for the organization of the final repetition and certification in the 11th grade [Text] / A.P. Carp. – M.: Enlightenment, 2005. – 79 p.
Kulanin, E.D. 3000 competitive problems in mathematics [Text] / E.D. Kulanin. – M.: Iris-press, 2007. – 624 p.
Leibson, K.L. Collection of practical tasks in mathematics [Text] / K.L. Leibson. – M.: Bustard, 2010. – 182 p.
Elbow, V.V. Problems with parameters and their solution. Trigonometry: equations, inequalities, systems. Grade 10 [Text] / V.V. Elbow. – M.: ARKTI, 2008. – 64 p.
Manova, A.N. Maths. Express tutor to prepare for the exam: account. allowance [Text] / A.N. Manova. - Rostov-on-Don: Phoenix, 2012. - 541 p.
Mordkovich, A.G. Algebra and beginning of mathematical analysis. 10-11 grades. Textbook for students of educational institutions [Text] / A.G. Mordkovich. – M.: Iris-press, 2009. – 201 p.
Novikov, A.I. Trigonometric functions, equations and inequalities [Text] / A.I. Novikov. - M.: FIZMATLIT, 2010. - 260 p.
Oganesyan, V.A. Methods of teaching mathematics in secondary school: General methodology. Proc. allowance for physics students. - mat. fak. ped. in-comrade. [Text] / V.A. Oganesyan. – M.: Enlightenment, 2006. – 368 p.
Olechnik, S.N. Equations and inequalities. Non-standard solution methods [Text] / S.N. Olekhnik. - M .: Publishing House Factorial, 1997. - 219 p.
Sevryukov, P.F. Trigonometric, exponential and logarithmic equations and inequalities [Text] / P.F. Sevryukov. – M.: National Education, 2008. – 352 p.
Sergeev, I.N. USE: 1000 tasks with answers and solutions in mathematics. All tasks of group C [Text] / I.N. Sergeev. – M.: Exam, 2012. – 301 p.
Sobolev, A.B. Elementary mathematics [Text] / A.B. Sobolev. - Yekaterinburg: GOU VPO USTU-UPI, 2005. - 81 p.
Fenko, L.M. The method of intervals in solving inequalities and studying functions [Text] / L.M. Fenko. – M.: Bustard, 2005. – 124 p.
Friedman, L.M. Theoretical foundations of the methodology of teaching mathematics [Text] / L.M. Friedman. - M .: Book house "LIBROKOM", 2009. - 248 p.
Attachment 1
Graphical interpretation of solutions to the simplest inequalities
Rice. one
Rice. 2
Fig.3
Fig.4
Fig.5
Fig.6
Fig.7
Fig.8
Appendix 2
Solutions to the simplest inequalities
