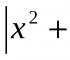Angles on the unit circle. Trigonometric circle

This article contains tables of sines, cosines, tangents and cotangents... First, we give a table of the main values ​​of trigonometric functions, that is, a table of sines, cosines, tangents and cotangents of angles 0, 30, 45, 60, 90, ..., 360 degrees ( 0, π / 6, π / 4, π / 3, π / 2, ..., 2π radian). After that, we will give a table of sines and cosines, as well as a table of tangents and cotangents of V.M. Bradis, and show how to use these tables when finding the values ​​of trigonometric functions.

Page navigation.

Table of sines, cosines, tangents and cotangents for angles 0, 30, 45, 60, 90, ... degrees

Bibliography.

• Algebra: Textbook. for 9 cl. wednesday school / Yu. N. Makarychev, N. G. Mindyuk, K. I. Neshkov, S. B. Suvorova; Ed. S. A. Telyakovsky.- M .: Education, 1990.- 272 p .: ill.- ISBN 5-09-002727-7
• Bashmakov M.I. Algebra and the beginning of analysis: Textbook. for 10-11 cl. wednesday shk. - 3rd ed. - M .: Education, 1993 .-- 351 p .: ill. - ISBN 5-09-004617-4.
• Algebra and the beginning of the analysis: Textbook. for 10-11 cl. general education. institutions / A. N. Kolmogorov, A. M. Abramov, Yu. P. Dudnitsyn and others; Ed. A. N. Kolmogorov. - 14th ed. - M .: Education, 2004. - 384 p .: ill. - ISBN 5-09-013651-3.
• Gusev V.A., Mordkovich A.G. Mathematics (manual for applicants to technical schools): Textbook. manual. - M .; Higher. shk., 1984.-351 p., ill.
• Bradis V.M. Four-digit mathematical tables: For general education. study. institutions. - 2nd ed. - M .: Bustard, 1999. - 96 p .: ill. ISBN 5-7107-2667-2

Trigonometric circle. Unit circle. Number circle. What it is?

Attention!
There are additional
materials in Special Section 555.
For those who are "not very ..."
And for those who are "very even ...")

Very often terms trigonometric circle, unit circle, number circle poorly understood by the student people. And completely in vain. These concepts are powerful and versatile helpers in all areas of trigonometry. In fact, this is a legal cheat sheet! I drew a trigonometric circle - and immediately saw the answers! Tempting? So let's learn, it's a sin not to use such a thing. Moreover, it is not difficult at all.

There are only three things you need to know to work successfully with the trigonometric circle.

If you like this site ...

By the way, I have a couple more interesting sites for you.)

You can practice solving examples and find out your level. Instant validation testing. Learning - with interest!)

you can get acquainted with functions and derivatives.

On the trigonometric circle, in addition to angles in degrees, we observe.

More about radians:

A radian is defined as the angular magnitude of an arc whose length is equal to its radius. Accordingly, since the circumference is , then it is obvious that a radian fits in a circle, that is,

1 rad ≈ 57.295779513 ° ≈ 57 ° 17′44.806 ″ ≈ 206265 ″.

Everyone knows that a radian is

So, for example, ah. That's how we learned to convert radians to angles.

Now on the contrary, let's convert degrees to radians.

Let's say we need to convert to radians. It will help us. We proceed as follows:

Since, radian, then fill in the table:

We train to find the values ​​of the sine and cosine in a circle

Let's further clarify the following.

Well, well, if we are asked to calculate, say, - there is usually no confusion here - everyone starts looking at the circle first.

And if they ask to calculate, for example, ... Many, suddenly, start not to understand where to look for this zero ... They often look for it at the origin. Why?

1) Let's agree once and for all! What comes after or is argument = angle, and the corners are located on the circle, don't look for them on the axles!(It's just that individual points fall on both the circle and the axis ...) And the values ​​of the sines and cosines themselves - we are looking for the axes!

2) And more! If we go from the "start" counterclock-wise(the main direction of traversing the trigonometric circle), then we postpone the positive values ​​of the angles, the values ​​of the angles increase when moving in this direction.

If we go from the "start" clockwise, then we postpone negative values ​​of the angles.

Example 1.

Find the value.

Decision:

We find it on the circle. We project a point onto the sine axis (that is, draw a perpendicular from the point to the sine axis (oh)).

We arrive at 0. Hence,.

Example 2.

Find the value.

Decision:

We find it on the circle (we go counterclockwise and more). We project a point onto the sine axis (and it already lies on the sinus axis).

We hit -1 on the sine axis.

Note that behind the point there are such points as (we could go to the point marked as, clockwise, which means a minus sign appears), and infinitely many others.

An analogy can be made:

Think of the trigonometric circle as a stadium treadmill.

After all, you can find yourself at the "Flag" point, starting from the start counterclockwise, running, say, 300 m. Or running, say, 100 m clockwise (we count the length of the track 400 m).

And also you can find yourself at the "Flag" point (after the "start"), having run, say, 700 m, 1100 m, 1500 m, etc. counterclockwise. You can get to the "Flag" point by running 500 m or 900 m, etc. clockwise from "start".

Expand the stadium treadmill mentally into a number line. Imagine where on this line there will be, for example, the values ​​300, 700, 1100, 1500, etc. We will see points on the number line, equidistant from each other. Let's roll back into a circle. The dots "stick together" into one.

So it is with the trigonometric circle. There are infinitely many others hidden behind each point.

Let's say angles,,, etc. are depicted by one point. And the values ​​of the sine, cosine in them, of course, coincide. (Did you notice that we added / subtracted or? This is the period for the sine and cosine function.)

Example 3.

Find the value.

Decision:

Let's translate for simplicity in degrees

(later, when you get used to the trigonometric circle, you will not need to convert radians to degrees):

We will move clockwise from the point Let's go half a circle () and more

We understand that the sine value coincides with the sine value and is equal to

Note, if we took, for example, or, etc., then we would get all the same sine value.

Example 4.

Find the value.

Decision:

However, we will not convert radians to degrees, as in the previous example.

That is, we need to go counterclockwise half a circle and another quarter of a half circle and project the resulting point onto the cosine axis (horizontal axis).

Example 5.

Find the value.

Decision:

How to postpone on the trigonometric circle?

If we pass or, yes, at least, we will still find ourselves at the point that we designated as "start". Therefore, you can immediately go to a point on the circle.

Example 6.

Find the value.

Decision:

We will find ourselves at the point (it will lead us to point zero anyway). We project the point of the circle onto the cosine axis (see trigonometric circle), we get into. I.e .

Trigonometric circle - in your hands

You already understood that the main thing is to remember the values ​​of the trigonometric functions of the first quarter. In the other quarters, everything is the same, you just need to follow the signs. And I hope you will not forget the "chain-ladder" of values ​​of trigonometric functions.

How to find tangent and cotangent values main corners.

After that, having got acquainted with the basic values ​​of tangent and cotangent, you can pass

On an empty circle template. Train!

Coordinates x points lying on the circle are equal to cos (θ), and the coordinates y correspond to sin (θ), where θ is the angle.

• If you find it difficult to remember this rule, just remember that in the pair (cos; sin) "sine is in last place".
• This rule can be deduced if we consider right-angled triangles and the definition of these trigonometric functions (sine of the angle is equal to the ratio the length of the opposite, and the cosine is the adjacent leg to the hypotenuse).

Write down the coordinates of the four points on the circle. A "unit circle" is a circle whose radius is equal to one. Use this to determine coordinates x and y at four points of intersection of the coordinate axes with the circle. Above, we have designated these points for clarity as "east", "north", "west" and "south", although they do not have an established name.

• "East" corresponds to a point with coordinates (1; 0) .
• "North" corresponds to a point with coordinates (0; 1) .
• "West" corresponds to a point with coordinates (-1; 0) .
• "South" corresponds to a point with coordinates (0; -1) .
• This is the same as a normal graph, so there is no need to memorize these values, just remember the basic principle.

Remember the coordinates of the points in the first quadrant. The first quadrant is located at the top right of the circle, where the coordinates x and y take positive values. These are the only coordinates you need to remember:

• point π / 6 has coordinates () ;
• point π / 4 has coordinates () ;
• point π / 3 has coordinates () ;
• note that the numerator only accepts three values. If you move in the positive direction (from left to right along the axis x and from bottom to top along the axis y), the numerator takes the values ​​1 → √2 → √3.

Draw straight lines and determine the coordinates of the points of their intersection with the circle. If you draw straight horizontal and vertical lines from the points of one quadrant, the second points of intersection of these lines with the circle will have coordinates x and y with the same absolute values, but different signs. In other words, you can draw horizontal and vertical lines from the points of the first quadrant and sign the points of intersection with the circle with the same coordinates, but at the same time leave room for the correct sign ("+" or "-") on the left.

• For example, you can draw a horizontal line between the points π / 3 and 2π / 3. Since the first point has coordinates ( 1 2, 3 2 (\ displaystyle (\ frac (1) (2)), (\ frac (\ sqrt (3)) (2)))), the coordinates of the second point will be (? 12 , ? 3 2 (\ displaystyle (\ frac (1) (2)),? (\ Frac (\ sqrt (3)) (2)))), where a question mark is placed instead of the "+" or "-" sign.
• Use the simplest method: note the denominators of the point coordinates in radians. All points with denominator 3 have the same absolute coordinate values. The same applies to points with denominators 4 and 6.

Use the symmetry rules to determine the sign of the coordinates. There are several ways to determine where to put the "-" sign:

• remember the basic rules for regular charts. Axis x negative on the left and positive on the right. Axis y negative below and positive above;
• start in the first quadrant and draw lines to other points. If the line crosses the axis y, coordinate x will change its sign. If the line crosses the axis x, the sign of the coordinate will change y;
• remember that in the first quadrant all functions are positive, in the second quadrant only the sine is positive, in the third quadrant only the tangent is positive, and in the fourth quadrant only the cosine is positive;
• whichever method you use, the first quadrant should be (+, +), the second (-, +), the third (-, -), and the fourth (+, -).

Check if you are wrong. Below is a complete list of coordinates of "special" points (except for four points on the coordinate axes), if you move along the unit circle counterclockwise. Remember that to determine all these values, it is enough to remember the coordinates of the points only in the first quadrant:

• first quadrant: ( 3 2, 1 2 (\ displaystyle (\ frac (\ sqrt (3)) (2)), (\ frac (1) (2)))); (2 2, 2 2 (\ displaystyle (\ frac (\ sqrt (2)) (2)), (\ frac (\ sqrt (2)) (2)))); (1 2, 3 2 (\ displaystyle (\ frac (1) (2)), (\ frac (\ sqrt (3)) (2))));
• second quadrant: ( - 1 2, 3 2 (\ displaystyle - (\ frac (1) (2)), (\ frac (\ sqrt (3)) (2)))); (- 2 2, 2 2 (\ displaystyle - (\ frac (\ sqrt (2)) (2)), (\ frac (\ sqrt (2)) (2)))); (- 3 2, 1 2 (\ displaystyle - (\ frac (\ sqrt (3)) (2)), (\ frac (1) (2))));
• third quadrant: ( - 3 2, - 1 2 (\ displaystyle - (\ frac (\ sqrt (3)) (2)), - (\ frac (1) (2)))); (- 2 2, - 2 2 (\ displaystyle - (\ frac (\ sqrt (2)) (2)), - (\ frac (\ sqrt (2)) (2)))); (- 1 2, - 3 2 (\ displaystyle - (\ frac (1) (2)), - (\ frac (\ sqrt (3)) (2))));
• fourth quadrant: ( 1 2, - 3 2 (\ displaystyle (\ frac (1) (2)), - (\ frac (\ sqrt (3)) (2)))); (2 2, - 2 2 (\ displaystyle (\ frac (\ sqrt (2)) (2)), - (\ frac (\ sqrt (2)) (2)))); (3 2, - 1 2 (\ displaystyle (\ frac (\ sqrt (3)) (2)), - (\ frac (1) (2)))).

Attention!
There are additional
materials in Special Section 555.
For those who are "not very ..."
And for those who are "very even ...")

Very often terms trigonometric circle, unit circle, number circle poorly understood by the student people. And completely in vain. These concepts are powerful and versatile helpers in all areas of trigonometry. In fact, this is a legal cheat sheet! Drew trigonometric circle- and immediately saw the answers! Tempting? So let's learn, it's a sin not to use such a thing. Moreover, it is not difficult at all.

There are only three things you need to know to work successfully with the trigonometric circle.

First. You need to know what sine, cosine, tangent and cotangent are as applied to a right-angled triangle. Follow the link who hasn't been there yet. Then everything will be clear here too.

Second. You need to know what it is trigonometric circle, unit circle, number circle. I will tell this right here and now.

Third. You need to know how to measure angles on a trigonometric circle, and what are the degree and radian measures of angles. This will be in the next lessons.

Everything. Having dealt with these three whales, we get reliable, trouble-free and perfectly legal cheat sheet for all trigonometry at once.

And then in school textbooks with this very trigonometric circle somehow not very ...

Let's start, little by little.

In the previous lesson, you learned that sine, cosine, tangent and cotangent (i.e. trigonometric functions) depend only on the angle. And do not depend on the lengths of the sides in right triangle... Hence an interesting question. Suppose we have such a corner. Let's call it angle β. The letter is beautiful.)

Since there is an angle, it must have trigonometric functions! Sine, say, or cotangent ... And where can I get them? There is no hypotenuse, no legs ...

How to determine trigonometric functions of an angle without right triangle? Problem ... We'll have to climb into the treasury of world knowledge again. To medieval people. They knew how to do everything ...

The first step is to take the coordinate plane. These are the most common coordinate axes, ОХ - horizontally, ОY - vertically. And ... nail one side of the corner to the positive OX semiaxis. The top of the corner, of course, is at point O. Let's beat hard so as not to tear it off! Leave the other side movable so that the angle can be changed. We will have a sliding corner. The end of the unattached side of the corner is denoted by a point BUT... We get the following picture:

So, the corner was attached. And where is its sine, where is the cosine? Calm down! Everything will be now.

Let's mark the coordinates of the point BUT on the axles. Hover your mouse over the picture and you will see everything. On OH this will be the point IN, on ОY - point FROM... It is clear that IN and FROM - these are some numbers. Point coordinates BUT.

So, number B will be the cosine of the angle β, and number C- his sine!

Why's that? The ancients taught us that sine and cosine are the relationship of the sides! Which do not depend on the lengths of the sides. And here we came up with the coordinates of the point ... But! Look at the triangle OAV... Rectangular, by the way ... According to the ancient definition, the cosine of the angle β is equal to the ratio of the adjacent leg to the hypotenuse. Those. OV / OA... Okay, we don’t mind. Moreover, the cosine and sine do not depend on the lengths of the sides. And this is generally great! This means that the lengths of the sides can be taken as desired. We have every right to take the length OA for a unit! It doesn't matter what. Even though a meter, even a kilometer, the sine still does not change. And in this case

Like this. If we carry out the same reasoning for the sine, we get that the sine of the angle β is equal to AB... But AB = OS... Hence,

It can be said quite simply. The sine of the angle β will be game room coordinate of point A, and cosine - xxx... The words are non-standard, but so much the better. It is remembered more reliably! And you need to remember this. It's hard to remember. Cosine - by x, sine - by game.

No, medieval people have not offended the ancients! Preserving the legacy! And the attitude of the parties was preserved, and the possibilities expanded enormously!

However, where trigonometric circle!? Where unit circle!? There was not a word about circles!

Right. But there is nothing left. Take the movable side OA and rotate it around point O for a full turn. What shape do you think point A will draw? Quite right! Circle! Here she is.

This is what it will be trigonometric circle.

Like this. Why is the circle trigonometric? Circle and circle ... The question is reasonable. Let me explain. Each point of the circle corresponds to two numbers. The X coordinate of this point and the Y coordinate of this point. And what coordinates do we have? Move the cursor over the drawing. Our coordinates are points B and C. That is. cosine and sine angle β. Those. trigonometric functions... Therefore, the circle is called trigonometric.

Remembering that OA= 1, and OA- radius, let's figure out that this is - and unit circle also.

And since sine and cosine are just some numbers- this trigonometric circle will also numeric circle.

Three terms in one bottle.)

In this topic, these concepts: trigonometric circle, unit circle and number circle- same. More broadly, unit circle Is any circle with a radius equal to one. Trigonometric circle- a practical term, just for working with the unit circle in trigonometry. What we are going to do now. Working with a trigonometric circle.

We have already completed the first half of the work. Draw a trigonometric circle using an angle (sounds great, doesn't it?).

Now let's do the second half of the job. Let's do the same, just the opposite. Let's go from the trigonometric circle to the corner.

Let us be given a unit circle. Those. just a circle drawn on a coordinate plane with a radius of one. Take arbitrary point A on the circle. Let's mark its coordinates by points B and C on the axes. As we recall, its coordinates are cosβ(by x) and sinβ(according to the game). And note the sine with cosine. We get the following picture:

All clear? Attention, question!

Where is β !? Where is the angle β, without which there is no sine and cosine !?

Hover the cursor over the picture, and ... here it is, here it is, angle β! It is its sine and cosine that are the coordinates of point A.

By the way, the nailed side of the corner is not drawn here. She is not needed in the previous drawings, just so, for understanding ... Angle always is measured from the positive direction of the OX axis. From the direction of the arrow.

And if point A is taken elsewhere? The circle is round ... Yes, please! Everywhere! Let's place, for example, point A in the second quarter, mark its coordinates, sine, cosine, as expected. Like this:

The most observant will notice that the sine of the angle β is positive (point FROM- on the positive semiaxis OY), but the cosine - negative! Point IN lies on the negative OX semiaxis.

Hover the cursor over the picture and see the angle β. The angle β is obtuse here. Which, by the way, is definitely not the case in a right-angled triangle. And in vain, perhaps, did we expand the possibilities?

Got the essence trigonometric circle? If you take a point anywhere in the circle, its coordinates will be the cosine and sine of the angle. The angle is measured from the positive direction of the OX axis and to the straight line connecting the center of coordinates with this very point on the circle.

That's all. It would be easier, but nowhere. By the way, my advice to you. When working with a trigonometric circle, draw not only points on the circle, but the corner itself! As in these pictures. It will be clearer.

You will constantly have to draw this circle in trigonometry. This is not an obligation, this is the legal cheat sheet that is used smart people... Doubts? Then call me by memory signs of such expressions, for example: sin130 0, cos150 0, sin250 0, cos330 0? I'm not asking about cos1050 0 or sin (-145 0) ... About such angles in the next lesson is written.

And you won't find a clue anywhere. Only on the trigonometric circle. Draw exemplary angle in the correct quarter and we can immediately see where its sine and cosine fall. On the positive semiaxes, or negative. By the way, determining the signs of trigonometric functions is constantly required in a variety of tasks ...

Or else, purely for example ... Do you need, for example, to find out which is greater, sin130 0, or sin155 0? Try it, just figure it out ...

And we are smart, we will draw a trigonometric circle. And draw a corner on it about 130 degrees. Proceeding only from that that it is greater than 90 and less than 180 degrees. Focusing on an angle, not a circle! Where the moving side of the corner crosses the circle, it will cross there. We mark the game coordinate of the intersection point. This will be sin130 0. Like in this picture:

And then, here, let's draw an angle of 155 degrees. Let's roughly draw, knowing that it is more than 130 degrees. And less than 180. Note also its sine. Hover your cursor over the picture, you will see everything. So what, which sine is bigger? It's really hard to make a mistake here! Of course sin130 0 is greater than sin155 0!

Long? Yah?! Nobody requires you to carefully draw a picture and provide animation! You will work with this site, and for this task you will draw the following picture in 10 seconds:

Another will not understand what kind of scribbles, yes ... And you calmly and confidently give the correct answer! Although, neatness does not interfere ... Otherwise, you can draw such a "circle" that the opposite answer will turn out ...

This puzzle is just one example of the vast possibilities of the trigonometric circle. It is quite possible to master these possibilities. What we will do next.

More often than not, you will have to have with trigonometric functions in the usual, algebraic notation. Like sin45 0, tg (-3), cos (x + y) and so on. Without any pictures and trigonometric circles! You have to draw this very circle yourself. With your hands. If, of course, you want to easily and correctly solve trigonometry tasks. Including the most advanced ones. But don't worry too much. Already on this site, in trigonometry, I will provide you with drawing circles! And you will master this extremely useful technique. Definitely.

Let's summarize the lesson.

In this topic, we have smoothly moved from trigonometric functions of an angle in a right triangle to trigonometric functions any corner. To do this, we needed to master the concepts "trigonometric circle, unit circle, number circle". It is very useful.)

Here I talked about the trigonometric circle as applied to sine and cosine. But tangent and cotangent are also possible see on the circle! One movement of the pen, and you easily and correctly determine the sign of the tangent - the cotangent of any angle, solve trigonometric inequalities and generally shake those around you with your trigonometric abilities.)

If you are interested in such perspectives, you can visit the lesson "Tangent and Cotangent on the Trigonometric Circle" in Special Section 555.

What do angles of 1000 degrees look like? What do negative angles look like? What is the mysterious number "Pi" that you inevitably come across in any section of trigonometry? And how is this "Pi" attached to the corners? All this is in the following lessons.